With enormous developments in Space sciences overtaking us, it is important to refresh our understanding of orbital mechanics from a basic perspective. Agrim Arsh, a young amateur enthusiast provides an easy-to-understand piece.
The curiosity to learn about our place and purpose in the Universe prompted us to look at it through sceptical eyes, and understand it through the scientific method of observation and application. We made models to explain the occurrences that we observed and continually refined them to make them fit our observations and worldview.
The earliest models of the Universe known to us were those proposed by ancient astronomers, including those in Greece, India, and China. With time, the models of the Universe have changed much in acceptance and prominence— from the Platonic Geocentrism that saw major additions from Eudoxus and Hipparchus, to the Ptolemaic model (Plato’s geocentric model with refinements), after a lengthy period, the Copernican Heliocentric Model and finally, our present models of the Universe, the most widely accepted currently being the ∧CDM Model (Lambda cold dark matter model) where ∧ refers to the cosmological constant, a constant coming into the model from the Einstein Field Equations.
But to understand astronomy, we must first understand the motions of the Earth. And as for every motion, we must choose a reference frame (observer) for our motion. So let’s understand the motions of the Earth concerning certain reference frames.
Relative to the Sun, there are three main motions of the Earth— Revolution (orbital motion), Rotation (rigid-body motion), and Precession (motion of the axis of rotation). The motion of the Earth is very much similar to the motion of a top always acted upon by a normal force.
Revolution
Revolution refers to the actual displacing motion of the Earth around the Sun. The path followed by the Earth is an ellipse, with the Sun situated at one of the foci. Amongst other properties of an ellipse is the fact that the sum of the distances of any point on the ellipse from the two foci is constant.
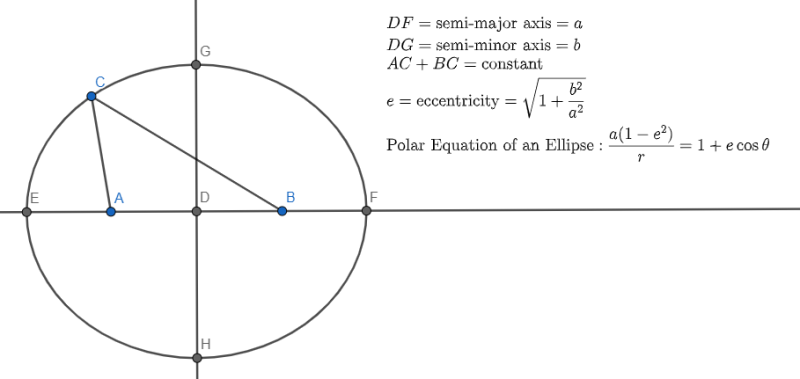
Image: All (that is needed) about ellipses (made with Geogebra)
If the Sun is placed at A, then the distance of the planet ranges from AF to AE. The planet is farthest from the Sun at F. This point is called the ‘Aphelion’, while the point E where it is closest to the Sun is called the ‘Perihelion’ (for any general body, these points are called ‘Periapsis’ and ‘Apoapsis’ and for the Moon, they are called ‘Perigee’ and ‘Apogee’ respectively).
[Proof¹ for the mathematically-inclined reader (requires calculus):
We can write the force equation for the planet in its orbit as:

and in calculus form:

We can eliminate ω with the help of the equation obtained from the conservation of angular momentum. Henceforth, we obtain:
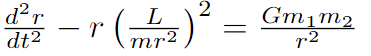
This second-order differential equation can be solved by substituting u for 1/r and ɵ (angle) for t:
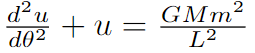
As you might know, the solution of this kind of differential equation is of the form A sin ɵ + C for some parameters. Similarly, this equation yields:

where A is a parameter depending on the initial conditions. This equation satisfies the polar equation of an ellipse (see image on ellipse). This proves the orbital path of planets to be an ellipse. ]
This law was put forth by Kepler (proof was given by Newton) and is known as Kepler’s First Law of Planetary Motion. Kepler’s Second Law states that the line separation vector between the Sun and the planet sweeps equal areas in equal intervals of time, i.e.

Kepler’s Third Law states that the square of the time of revolution of a planet is directly proportional to the cube of its semi-major axis:

Choosing T in years (specifically, Julian Year; 1 Julian year=(exactly) 365.25 days), and R in AU (astronomical unit, defined as the distance between the Earth and Sun; approximately 149.6 million km), the constant of proportionality equals 1.
Problem (for the mathematically inclined): Prove the above two results.
The Earth’s eccentricity is quite small (about 0.0167) and very slightly variable, as the Earth is affected by the gravitational forces of other Solar System Objects in its orbit. The plane of the orbit of a planet around the Sun is known as the Orbital Plane and is not necessarily the same for all the planets and moons (in fact, it is extremely rarely the case so). For example, the orbital plane of the moon is inclined to that of the Earth at about 5°.
All the planets revolve around the Sun in a counterclockwise direction when viewed from the top because this was just the way remnant material and accretion disk from the solar nebula started spinning, by chance, during the birth of the Solar System.
Earth’s one tropical year, defined as the time taken by the Earth to reach the same spot in its orbit again, is 365 days 5 hours 48 minutes 46 seconds with minute variations.
Rotation
Rotation is the rigid-body motion of the Earth, i.e. its motion on its axis. The plane passing normally to the line connecting the Earth’s pole and through its center of rotation, the Equatorial Plane, is inclined to its Orbital Plane at an angle of about 23.4° which is responsible for the change in seasons on the surface depending on which side of the Earth faces the Sun.
So what’s the Earth’s period of rotation? 24 hours? Some might be a “bit more accurate” and say 23 hours 56 minutes 4 seconds. In fact, both are right, depending upon the definition:

Image: Completion of a Sidereal Day (pos. 2) compared to the completion of a Solar Day (pos. 3) (in source: Wikimedia Commons, licensed under CC BY-SA 3.0
1 Sidereal Day is defined to be the time taken for a general star to come to a specific position in the night sky again and is equal to approximately 23 hours and 56 minutes. 1 Solar Day, on the other hand, is defined to be the time taken for the Sun to come to a specific position in the sky again and is equal to about 24 hours. How? Let’s not forget to count in the effect of the Earth’s revolution when calculating the period for a day with the help of the Sun. We know the Earth takes about 365 days to complete a revolution (360°) around the Sun. This means that in a day, it has covered (360°/365≈0.986°) in its orbit. This means that the Sun needs to cover 0.986° more to be at the same position it was the day before. Therefore, the difference in time between 1 Solar Day and 1 Sidereal Day = (0.986°/360°)×24 hours ≈ 0.066 hours ≈ 4 minutes. This is what we see as the “extra daytime” in 24 hours of the solar day. It is due to this difference in solar and sidereal timekeeping that we see the appearance of zodiac changes in the night sky.
Furthermore, the length of the Solar Day is also variable! Remember that Second Law given by Kepler in the previous section. That implies that the Earth revolves faster when it is closer to the Sun (near the Perihelion), implying that the Sun needs to cover slightly more than 0.986° to complete 1 Solar Day, implying that the length of the Solar Day is even larger. The opposite is true in the case of Aphelion and the length of the Solar Day reduces. Presently, the Perihelion takes place on about the 3rd of January. Thus, the actual longest day for the whole of Earth takes place in Northern Hemisphere’s winters (the Southern Hemisphere residents enjoy more daylight than the Northerners!).
The Earth spins in the same direction it revolves, i.e. counter-clockwise as seen from the top. This is what is known as Prograde motion. In fact, most of the planets in the Solar System are in a prograde motion. Why? As told previously, the solar accretion, by chance, came to be spinning counter-clockwise. Thus, when the planets were formed by the lumping of this accretion material, they had to conserve their angular momenta. This sped up their rotation (just like a skater speeds up when drawing his arms together) in the prograde direction. Uranus and Venus are the only planets that spin retrograde, i.e. in the opposite direction to their revolving directions. The cause for this is thought to be collisions of these planets early on in their lives (you see, they had a pretty ‘rocky’ start), which impacted their angular momenta adversely and changed their directions of motion.
Another interesting corollary of celestial motion is that the period of rotation of some satellites might be in sync with their period of revolution. This is what is known as ‘tidal locking’. This is an effect of the barycentre’s (center of mass’) gravitational effect on the satellite.

Image: Mechanism of Tidal Locking— The central body generates a gravitational torque on the satellite that stresses mass along the orbital direction of the satellite. The changed angular momentum as a result of this gravitational torque synchronizes the rotation period and the revolution period in a 1:1 ratio (for orbits with low eccentricity). For periods with a large tidal effect and eccentricity of orbits, the synchronization is not in a 1:1 ratio, but other simple ratios like 3:2 are more stable, for example in the case of Mercury’s tidal locking around the Sun. The given image shows the net torques about the surface of the body (in Public Domain.
You might have heard that we see only one side of the Moon. This is a result of the Tidal Locking of the Moon around the Earth in a 1:1 ratio. With repeated observation, about 59% of the Moon is visible from the Earth, due to the effects of liberation (change in perspective from different locations).
Precession and Fine Adjustments
A lot of the figures above are reported to be only a neat approximation. The reason for this is that these figures might undergo a slow periodic change. The cause of this change is the third type of motion of the Earth, Precession. Axial Precession is defined as the circular motion of the Earth’s axis of rotation. It is caused because of the gravitational effects of the Sun and the Moon on the equatorial bulge of the Earth (the shape of Earth). The Earth ‘precesses’ once in about 25,772 years. It is responsible for a large number of fine effects in the motions of the Earth.
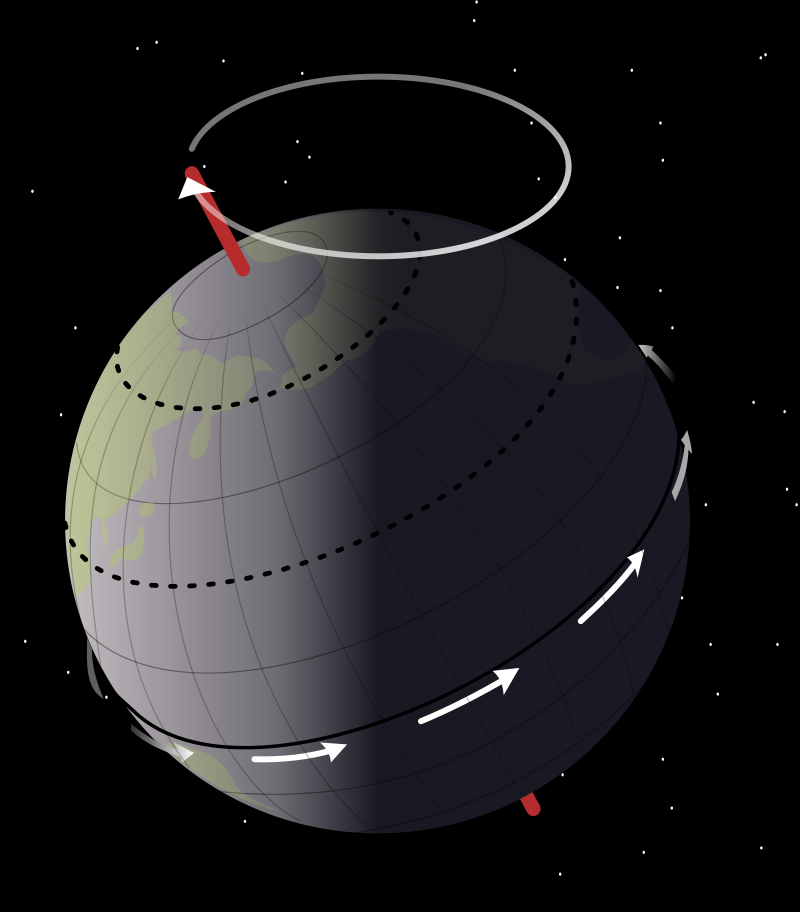
Image: Axial Precession of the Earth (in Public Domain)
Because of the Precession of the Earth, the time of the seasons has been slowly changing. If the currently accepted Calendar Year would have been equivalent to the Solar Year, then in some time, Christmas in the Northern Hemisphere would have been celebrated in the heat of summer in July. This is known as the ‘Precession of the Equinoxes’. However, this situation is avoided because the Gregorian Calendar is based on the seasons themselves. However, precession will have a clear effect on the climate of the Earth. As of the present, the perihelion lies during the winter of the Northern Hemisphere, making them less severe and the summer of the Southern Hemisphere more severe. However, in some time, this will reverse, resulting in more severe winters in the Northern Hemisphere.
Axial Precession also changes the star alignment on the Earth. Our pole star will change from Polaris to Vega and then to Thuban before coming back to Polaris within the next 26,000 years.
There is also an Apsidal Precession of the Earth— changes in the shape of its elliptical orbit (its eccentricity) due to fine adjustments (gravitational effects of other planets), resulting in an approximately 112,000-year cycle.
This is not all. The precessional trajectory of the Earth is also not a perfect circle, but a kind of wavy one. This causes a periodic change in the axial tilt of the Earth. The resulting motion of ‘wavy precession’ is what is known as Nutation.

Thus, quoting my previous sentence, the Earth’s motion is pretty much like the spinning of a top— rotation, precession, and nutation. However, there are also a large number of very, very fine adjustments in these periods, as an exactly precise measurement requires a solution to the ‘Three-Body Problem’ (more on it in later articles).
So this was pretty much everything about the motion(s) of the Earth from the Reference frame of the Sun, and as a rigid body on its own. In the next article, we will take this idea further and will look through the motions of other celestial bodies with different frames of reference.
All images used are in the public domain otherwise stated.
This article is published earlier in medium.com
Feature Image: Clouds over the Southern Pacific Ocean taken from Sun (in Public Domain) – medium.com






